
As you can see, every term is actually just a different power of 3:ģ 0, 3 1, 3 2, 3 3, 3 4, 3 5, … Who wants to be a Millionaire?
Arithmetic and geometric sequences pdf#
insurance, LIC AAO and for all types of banking exams with pdf > 12/3. Schoology Quiz : Level 2 - Arithmetic & Geometric Sequences 4. This sequence of numbers has a special name: the powers of 3. Identifying an Arithmetic Sequence Is the sequence of numbers an arithmetic sequence 0, 2, 4, 6, 8, 10, The number 0 is the first term, 2 is the second term, 4 is the third term etc. In the 10th step, you would reach 19,683 new ones, and after 22 steps you would have reached more people than are currently alive on Earth. The number of people increases incredibly quickly. Using the explicit formula for geometric sequences, we can work out how many new people are affected at any step:

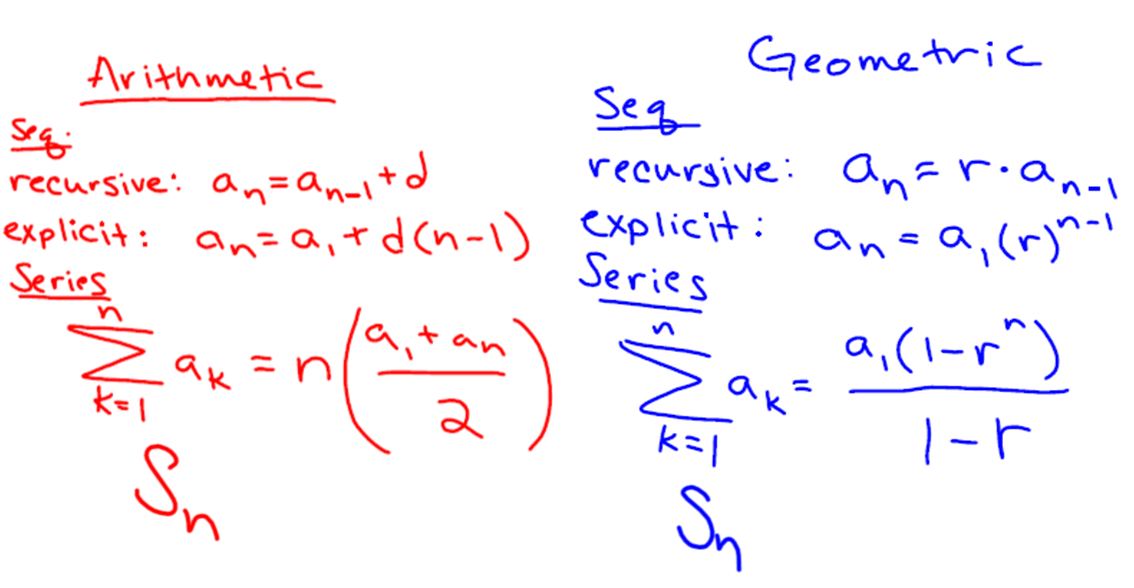
In contrast, a geometric sequence is one where each term equals the one before it multiplied by a certain value. For example: 5, 10, 15, 20, Each term in this sequence equals the term before it with 5 added on. A sequence is a list of numbers or objects, called terms, in a certain order.
Arithmetic and geometric sequences plus#
Notice how the number of people at every step forms a geometric sequence arithmetic sequence triangle number, with common ratio : An arithmetic series is one where each term is equal the one before it plus some number. Comparing Arithmetic, Geometric, Quadratic Sequences. The essence of Trevor’s idea is that, if everyone “pays it forward”, a single person can have a huge impact on the world: In 1682, the astronomer Edmond Halley observed an unusual phenomenon: a glowing white object with a long tail that moved across the night sky. In an arithmetic sequence, the new term is obtained by adding or subtracting a fixed value to/from the preceding term.

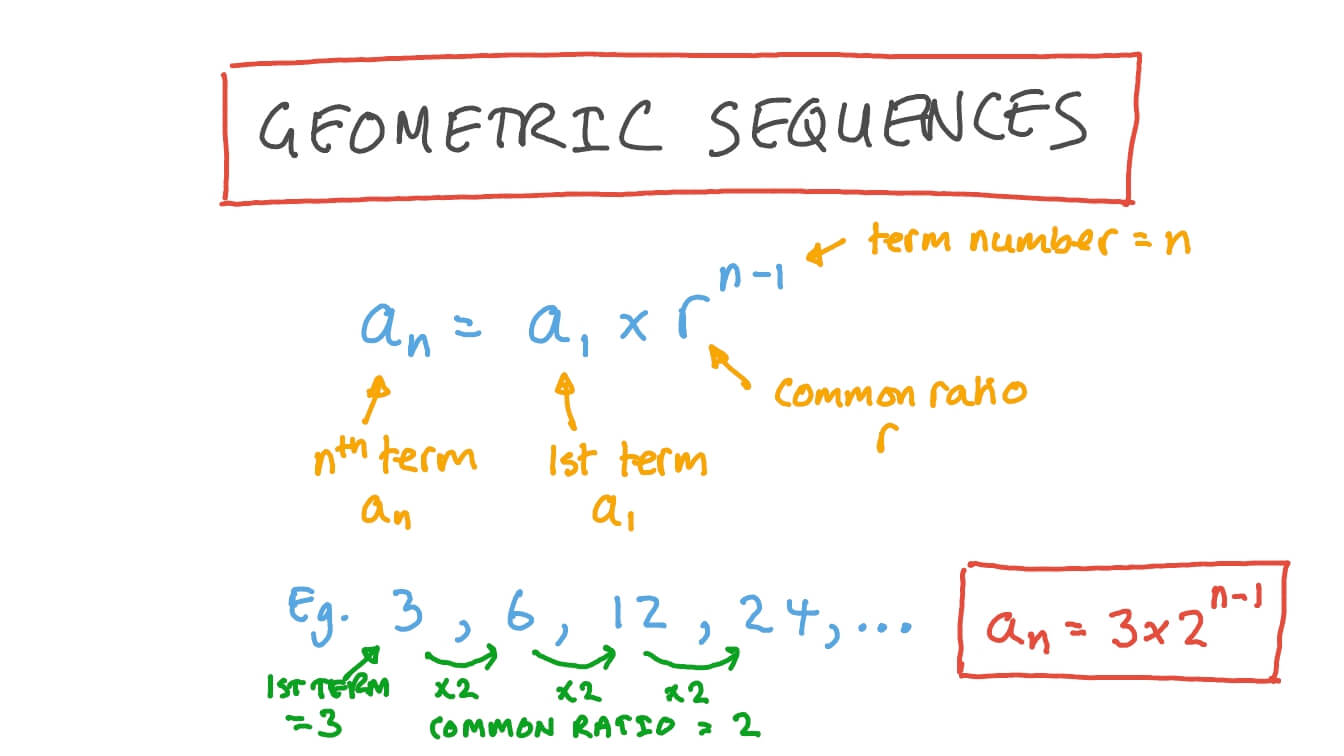
Extract from “Pay It Forward” (2000), © Warner Bros. Find a rule for this arithmetic sequence. In such a series, a 1 is called the first term, and the constant term r is called the common ratio of G.P. An arithmetic sequence has a common difference of 9 and a (41) 25. If we want to find any term (as the n term) in the arithmetic sequence, we can. An arithmetic sequence is a list of numbers with a defined pattern. For instance, the sequenceĠ 1, 1 2, 2 4, 3 8, 4 16, 5 32, ⋯. In a more general way, a sequence a 1, a 2, a 3 a n can be called a geometric progression if a n+1 a n. Arithmetic and Geometric Sequences Formulas and Examples Arithmetic sequences. If the common ratio of all terms in a sequence is the same, it is geometric sequence. In 1682, the astronomer Edmond Halley observed an unusual phenomenon: a glowing white object with a long tail that moved across the night sky. Arithmetico-geometric sequences arise in various applications, such as the computation of expected values in probability theory. How do you know if a sequence is arithmetic or geometric Ans: The following pointers are to be noted while determining a sequence is arithmetic or geometric: If the common difference of all terms in a sequence is the same, the sequence is arithmetic. Put plainly, the nth term of an arithmetico-geometric sequence is the product of the nth term of an arithmetic sequenceĪnd the nth term of a geometric one. In mathematics, arithmetico-geometric sequence is the result of term-by-term multiplication of a geometric progression with the corresponding terms of an arithmetic progression.


 0 kommentar(er)
0 kommentar(er)
